Nonadiabatic Dynamics
The Foundations of Surface Hopping
Beyond Marcus theory, our group is very interested in modeling general nondiabatic phenomena, including electron and energy transfer, electronic relaxation, and molecular conduction with vibrations. To that end, we have now systematically analyzed the origins of Tully's
fewest switches surface hopping algorithm (FSSH) algorithm.
Surface hopping began as a guessed algorithm. Originally Preston and Tully proposed rules for moving nuclei between different potential energy surfaces when one encounters a crossing. Later, Tully proposed a fewest switches surface hopping (FSSH) algorithm with a continuous probability to hop based on the time step. In our research group, we are interested in understanding the foundations of surface hopping, going back to the Schrodinger equation. In particular, we have been able to show that--provided decoherence is properly treated and provided we work with a few more approximations--the FSSH algorithm approximately follows that Quantum Classical Liouville Equation. This work has shed new light on where surface hopping comes from, what the limitations are, how we should interpret the algorithm, and how can we use the algorithm most robustly.
As a result of our ``derivation,'' we now understand
that surface hopping is an approximate means to propagate a quantum-classical density matrix (which we can now construct).
Admittedly, many questions still remain as to the formal foundations of surface hopping at low temperature, however.
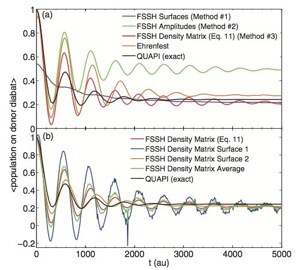
36. Landry, Falk, and Subotnik. The correct interpretation of surface hopping trajectories: How to calculate electronic Properties. J. Chem. Phys. (Communications) 139, 211101 (2013) [PDF]
35. Subotnik, Ouyang and Landry. Can we derive Tully's surface-hopping algorithm from the semiclassical quantum Liouville equation? Almost, but only with decoherence. . Chem. Phys. 139, 214107 (2013) [PDF]
Decoherence
Beyond understanding the origins of surface hopping, our research group has also been able to derive a cheap and simple means to incorporate decoherence into the FSSH algorithm. Long ago, key research groups in dynamics (Rossky, Truhlar, Hammes-Schiffer et al) pointed out that FSSH suffered from over-coherence, in the sense that nuclear wave packets on different electronic surfaces are not ever allowed to separate, or "decohere". The results can be fictitious oscillations in branching ratios or incorrect long-time dynamics. Many flavors of decoherence have been proposed over the last twenty years, but always with some empirical factor. We have now implemented a parameter-free decoherence correction to surface-hopping dynamics (titled augmented A-FSSH), based on the premise of integrating the differences in forces between adiabatic surfaces. Thus far, it has led to large improvements in accuracy for many problems (especially when friction is not overdamped).
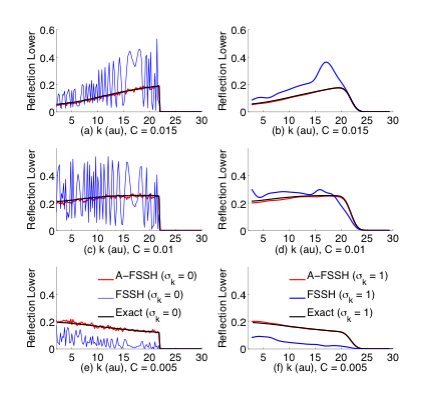
19. Subotnik and Shenvi. A New Approach to Decoherence and Momentum Rescaling in the Surface Hopping Algorithm J. Chem. Phys. 134, 024105 (2011) [PDF]
30. Landry and Subotnik. How to recover Marcus theory with fewest switches surface hopping: Add just a touch of decoherence J. Chem. Phys. 137, 22A513 (2012) [PDF]
Moreover, we have shown that an incorrect treatment of decoherence can lead to the wrong scaling for the Marcus rate of electron transfer theory:
27. Landry and Subotnik. Standard Surface Hopping Predicts Incorrect Scaling for Marcus’ Golden-Rule Rate: The Decoherence Problem Cannot Be Ignored J. Chem. Phys. Communication 135, 191101 (2011) [PDF]
Nonadiabatic Chemical Rate Theory
For long-lived nonadiabatic proceses, direct dynamics are not feasible and one should use transition state theory. When applying surface hopping techniques to nonadiabatic transition state theory, there is the inevitable problem of time irreversibility. FSSH dynamics are not time reversible, which could in principle lead to difficulties. This problem was studied previously by Hammes-Schiffer and Tully. One thrust of our research has been to re-examine this problem, now with the aim of including decoherence (which would make the problem even harder). We find that very good rates of reaction can be computed simply by propagation along the lower adiabatic state going backwards in time; simultaenously, one allows branching going forward in time to recover the correct transmission ratio. While this model for rate theory does not exactly satisfy detailed balance, it does pretty well in practice.
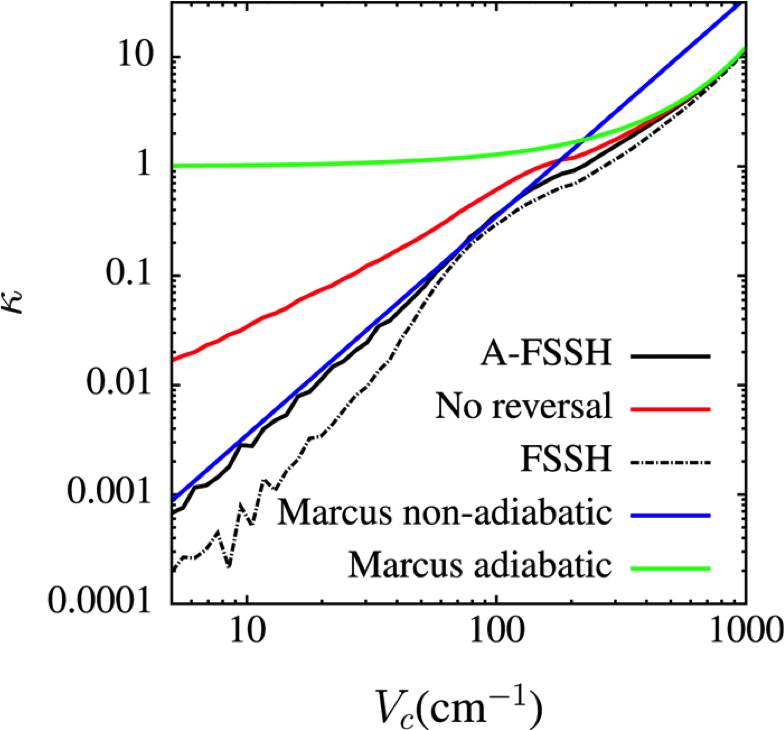
62. A. Jain and J.E. Subotnik. "Surface hopping, transition state theory and decoherence 2: Thermal rate constants and detailed balance.
J. Chem. Phys.
143, 134107 (2015)
[PDF]
link
Marcus Theory
One of our first key results was that, without decoherence, Tully's FSSH does not agree with Marcus theory. Interestingly, instead of V2 scaling, the algorithm often predicts V scaling.
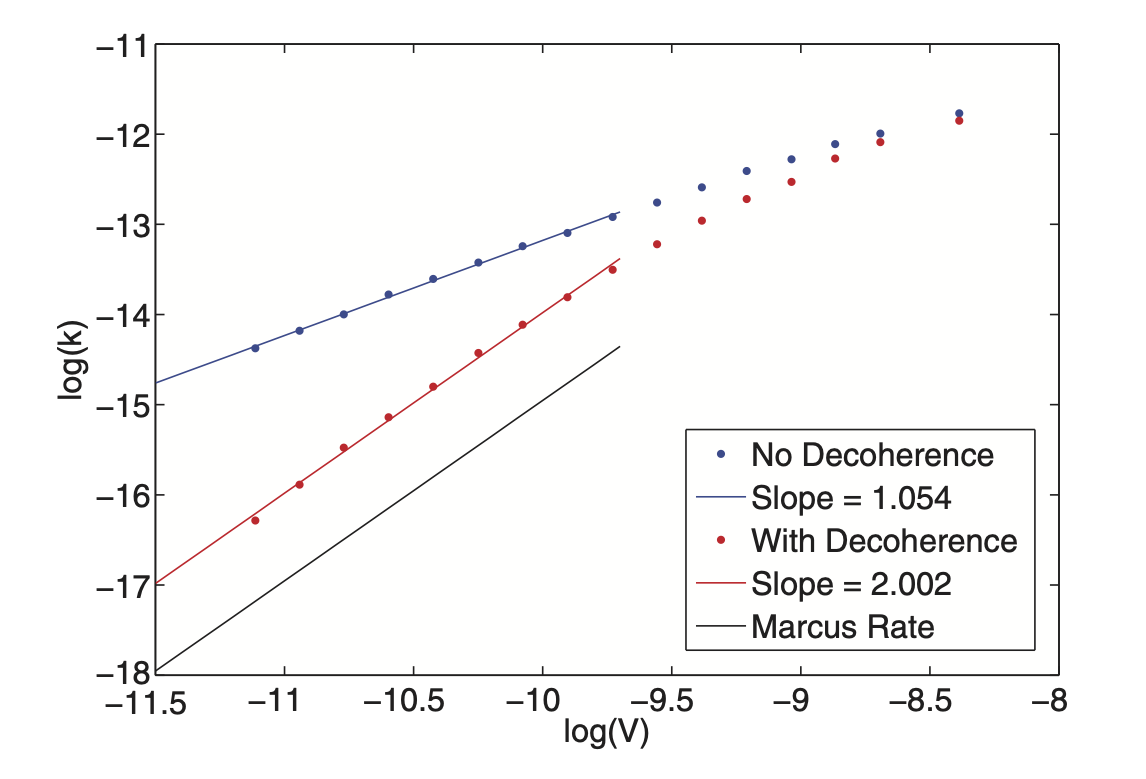
27. Landry and Subotnik. "Communication: Standard Surface Hopping Predicts Incorrect Scaling for Marcus’ Golden-Rule Rate: The Decoherence Problem Cannot Be Ignored."
J. Chem. Phys.
135, 191101 (2011)
[PDF]
link